Tractate four of Guarino Guarini’s treatise, Architettura civile (1735), deals entirely with orthographic projection. In “Observation Nine” of chapter three in the fourth tractate, Guarini describes the process of obtaining the drawings for a vault that intersects a cylinder at an oblique angle. He begins by describing the oblique section of a cylinder as an ellipse, referencing Euclid. He goes on to explain that since we can measure the height and breadth of the ellipse in the orthographic drawings, we have the information to construct the ellipse that is the section through the cylinder at the plane of intersection. He also provides a template for the ellipse and instructs the reader to make a drawing instrument out of “strong card” using the template as a guide. Guarini utilized an argument from Euclid to create an instrument that draws curves specific to the angle of incidence between a cylinder and an orthographic projection.
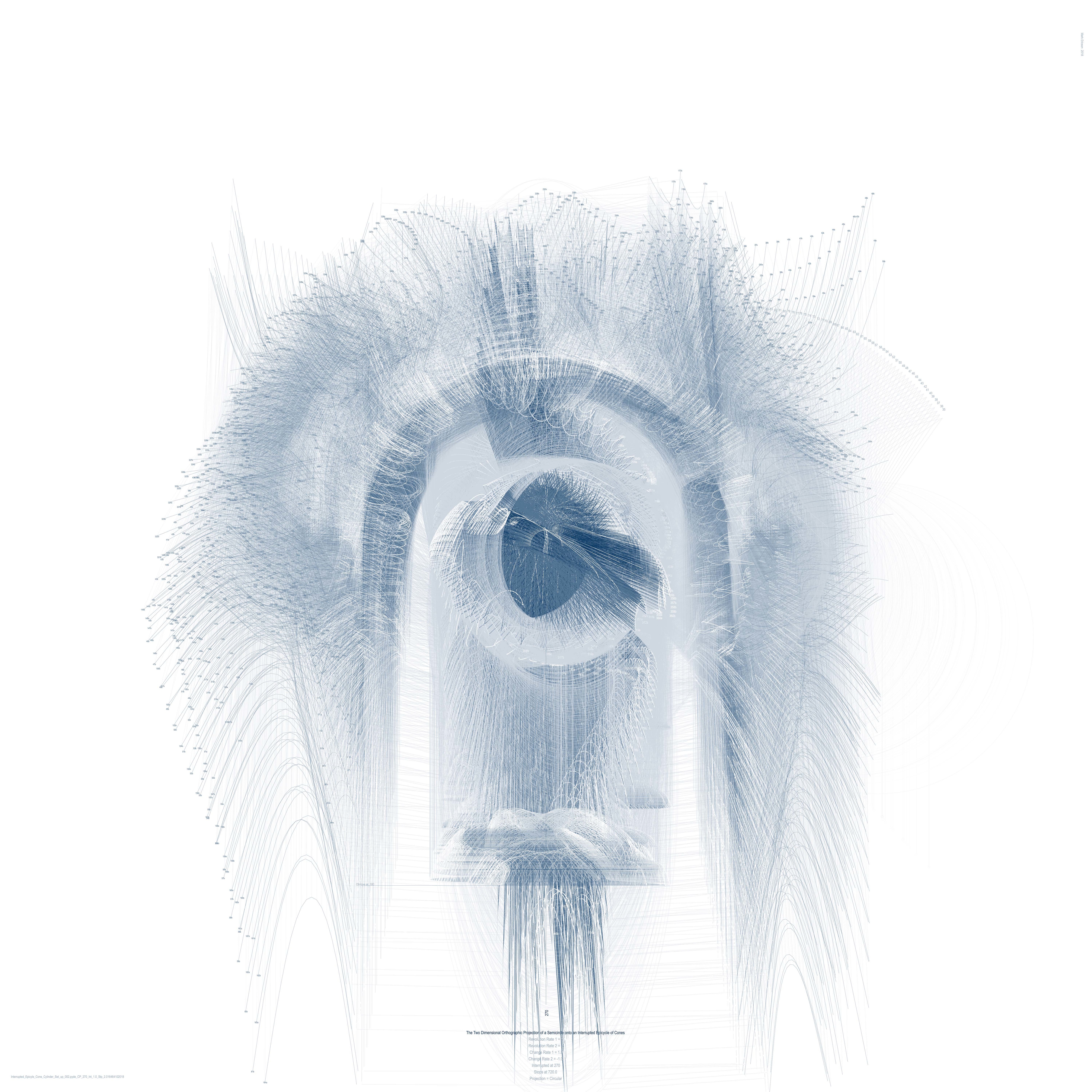
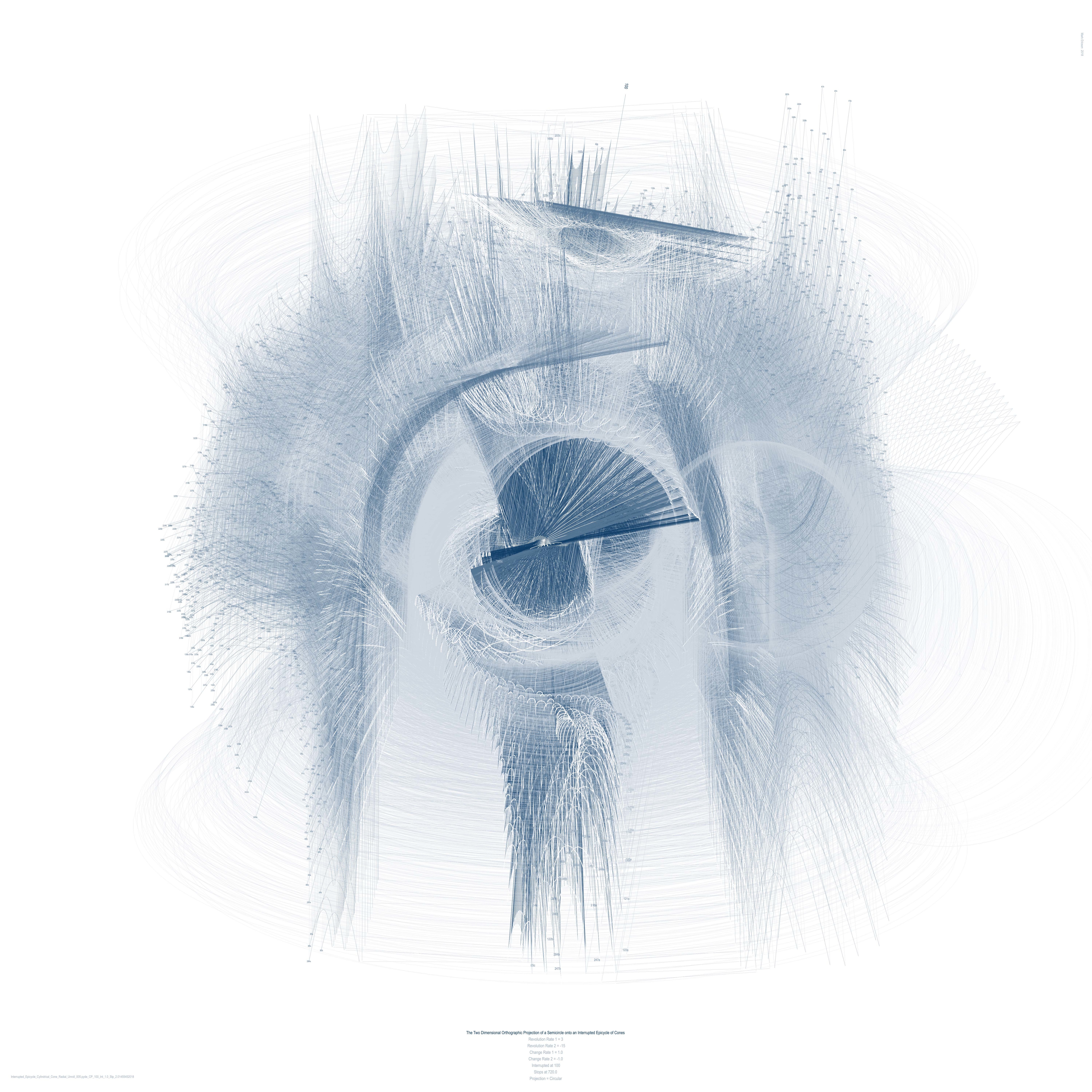
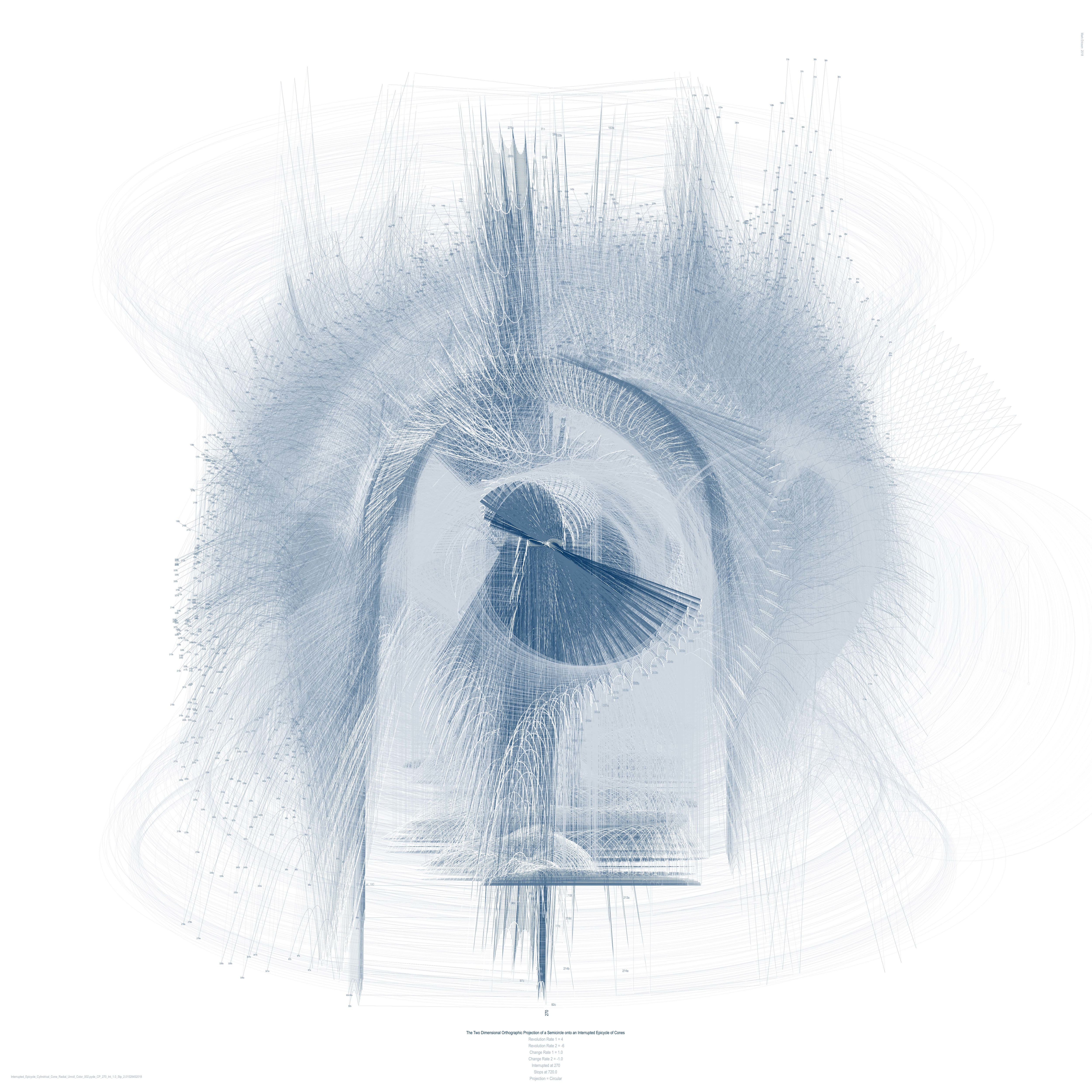
The drawings included in this page, are part of series that translates the written and drawn instructions from the fourth tractate of Architettura civile, into a set of repeatable procedures that vary over time. These drawings use the techniques of “Observation Nine” to orthographically project a semicircle onto cones moving about the minor and major orbits of two epicycles and one hypocycloid. The cones vary in height, orientation, and radius over time, producing differing conic sections. Whereas Guarini produced a single static template, this drawing utilizes the same techniques to produce a set variable conic sections. Importantly, like Guarini’s work this drawing is confined to the use of points, lines, circles, and ellipses on a two-dimensional plane. There are no three-dimensional objects, surfaces, or curves of any kind. Each drawing is an orthographic projection.
This particular series unrolls each projection perpendicular to the rotating centerline of each projection. This results in a radially unfolding the individual elevations.
©Mark Ericson 2018