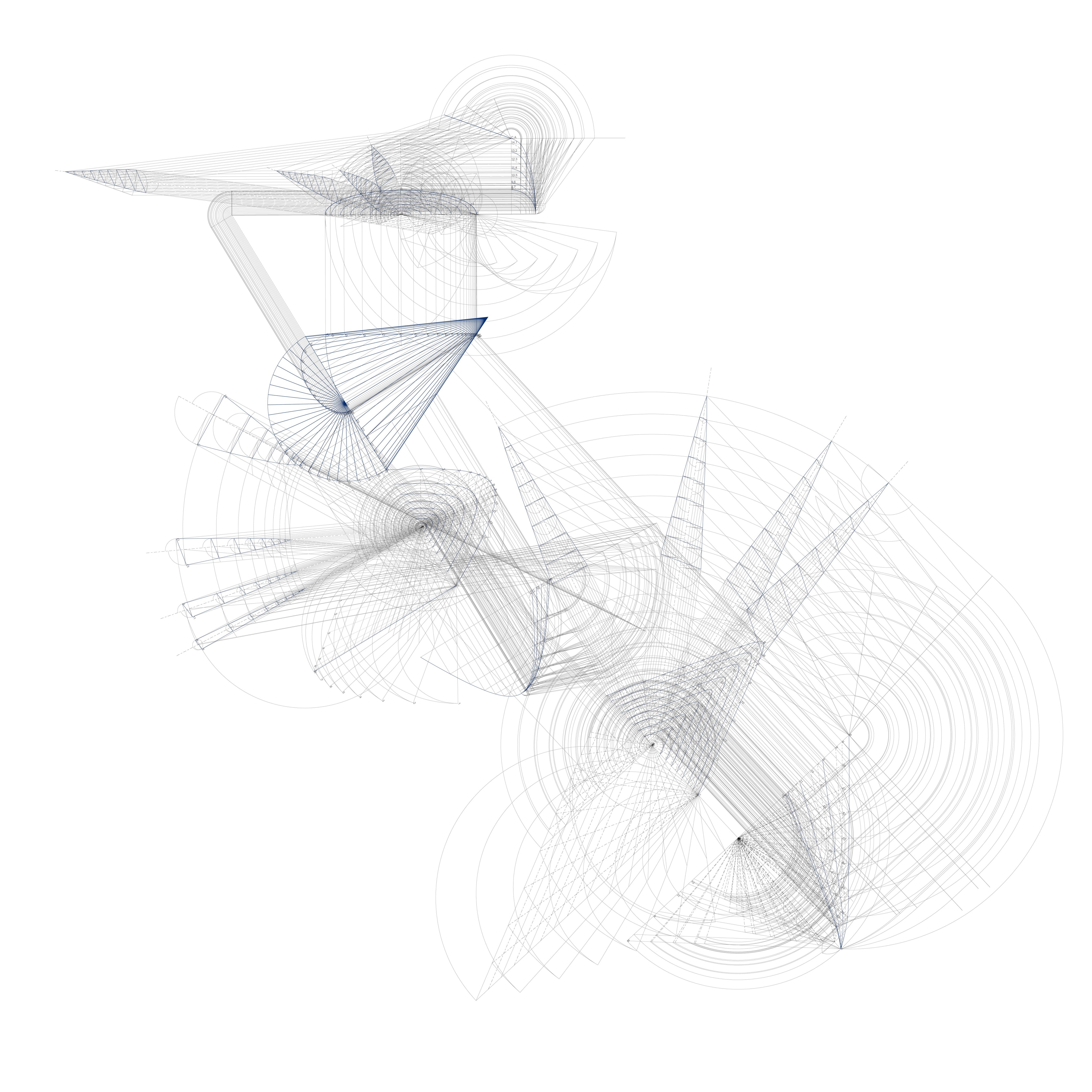

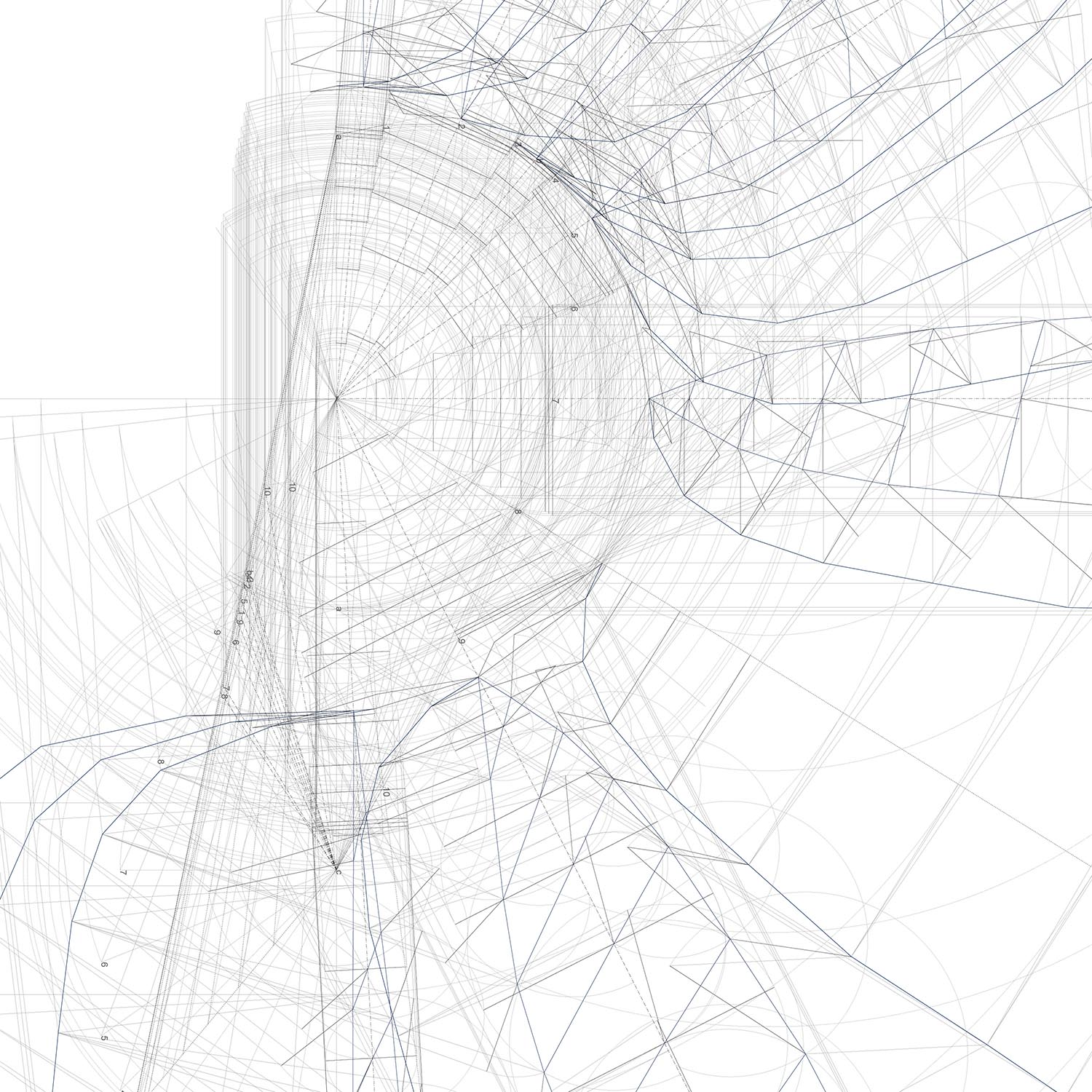
Euclid’s Wedge
Euclid’s Elements (300 B.C.) does not contain a single reference to architectural drawing. And yet regardless of its absence from the text, the architectural practice of graphically representing and geometrically calculating the shape of objects and buildings through orthographic projection is based largely on Euclidean propositions. While orthography is the most obvious architectural derivative of Euclid, other drawing practices also emerged from this text that are less discussed, but perhaps more relevant to contemporary discourse. A clear example of this is the drawing practice that calculated the shape of the stones that composed vaults during the 16th and 17th. Orthography and the use of “views” and sections associated with this practice, is not sufficient to deal with the wide variety of formal and spatial conditions that arise in the practice of vault design. The greatest difficulty that orthography faces arises in the construction of curved vaults that are non-spherical. Spherical vaults, contain circular sections that are identical through both ninety and one-hundred and eighty degrees of rotation. This allows for side and section views to be orthographically constructed from front views without additional information. In contrast, Ellipsoid, Hyperboloid, and Paraboloid vaults do not share this characteristic. The section in each of these figures varies through ninety degrees of rotation. Stated simply, an ellipsoid cannot be generated from an ellipse through orthography alone. An additional means of drawing is required. A drawing practice that has much more to do with calculating than it does with visualizing. This ongoing research has involved the construction a series of research drawings that attempt to both understand the operative logic of Euclid’s wedge as well as speculate on alternative constructions.